삼각 함수의 덧셈 정리의 두 가지 증명입니다.
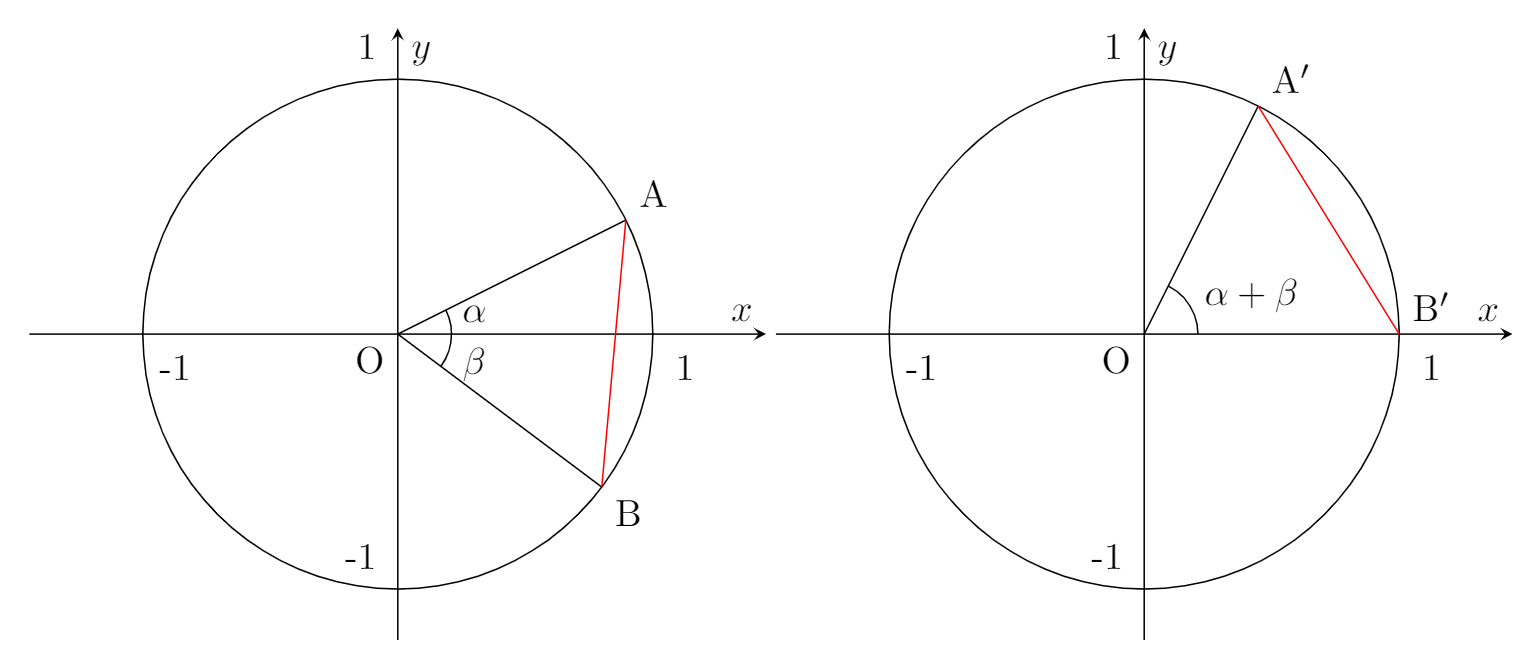
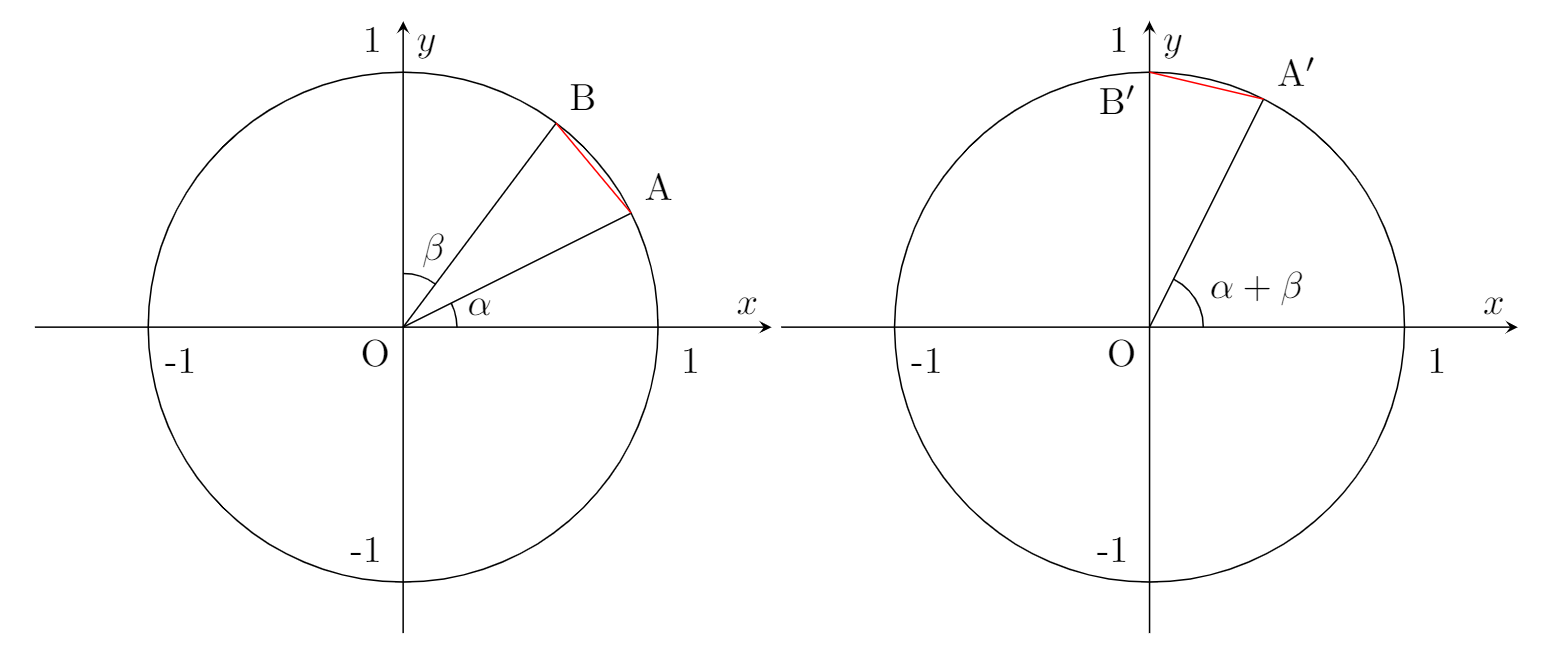
위 두 그림에서 빨간색으로 표시된 \(\overline{\mathrm{AB}}=\overline{\mathrm{A'B'}}\)임을 이용합니다. \(\triangle\mathrm{OAB}\equiv\triangle\mathrm{OA'B'}\) (SAS 합동)이기 때문입니다.
$$\mathrm{A}(\cos\alpha,\sin\alpha),\,\mathrm{B}(\cos\beta,\sin\beta)$$
$$\begin{aligned}\overline{\mathrm{AB}}^2&=(\cos\alpha-\cos\beta)^2+(\sin\alpha+\sin\beta)^2\\&=2-2\cos\alpha\cos\beta+2\sin\alpha\sin\beta\end{aligned}$$
$$\mathrm{A'}(\cos(\alpha+\beta),\sin(\alpha+\beta)),\,\mathrm{B'}(1,0)$$
$$\begin{aligned}\overline{\mathrm{A'B'}}^2&=(\cos(\alpha+\beta)-1)^2+\sin^2(\alpha+\beta)\\&=2-2\cos(\alpha+\beta)\end{aligned}$$
$$\therefore 2-2\cos\alpha\cos\beta+2\sin\alpha\sin\beta=2-2\cos(\alpha+\beta)$$
$$\therefore \cos(\alpha+\beta)=\cos\alpha\cos\beta-\sin\alpha\sin\beta$$
또, 위 두 그림에서 빨간색으로 표시된 \(\overline{\mathrm{AB}}=\overline{\mathrm{A'B'}}\)임을 이용합니다. 마찬가지로 \(\triangle\mathrm{OAB}\equiv\triangle\mathrm{OA'B'}\) (SAS 합동)이기 때문입니다.
$$\mathrm{A}(\cos\alpha,\sin\alpha),\,\mathrm{B}(\sin\beta,\cos\beta)$$
$$\begin{aligned}\overline{\mathrm{AB}}^2&=(\cos\alpha-\sin\beta)^2+(\sin\alpha-\cos\beta)^2\\&=2-2\cos\alpha\sin\beta-2\sin\alpha\cos\beta\end{aligned}$$
$$\mathrm{A'}(\cos(\alpha+\beta),\sin(\alpha+\beta)),\,\mathrm{B'}(0,1)$$
$$\begin{aligned}\overline{\mathrm{A'B'}}^2&=\cos^2(\alpha+\beta)+(\sin(\alpha+\beta)-1)^2\\&=2-2\sin(\alpha+\beta)\end{aligned}$$
$$\therefore 2-2\cos\alpha\sin\beta-2\sin\alpha\cos\beta=2-2\sin(\alpha+\beta)$$
$$\therefore \sin(\alpha+\beta)=\sin\alpha\cos\beta+\cos\alpha\sin\beta$$
\(\tan\)에 대해서는 특별히 직관적인 방법을 찾지 못했습니다.
$$\tan\theta=\frac{\sin\theta}{\cos\theta}$$
임을 이용합니다.
$$\begin{aligned}\tan(\alpha+\beta)&=\frac{\sin(\alpha+\beta)}{\cos(\alpha+\beta)}\\&=\frac{\sin\alpha\cos\beta+\cos\alpha\sin\beta}{\cos\alpha\cos\beta-\sin\alpha\sin\beta}&\text{divide both sides by $\cos\alpha\cos\beta$}\\&=\frac{\tan\alpha+\tan\beta}{1-\tan\alpha\tan\beta}\end{aligned}$$
따라서,
$$\sin(\alpha+\beta)=\sin\alpha\cos\beta+\cos\alpha\sin\beta$$
$$\cos(\alpha+\beta)=\cos\alpha\cos\beta-\sin\alpha\sin\beta$$
$$\tan(\alpha+\beta)=\frac{\tan\alpha+\tan\beta}{1-\tan\alpha\tan\beta}$$
이때 증명 방법에 따라 \(\alpha\)와 \(\beta\)의 크기에 대해 일반적으로 위
이때, \(\sin\)과 \(\cos\)에 대한 덧셈 정리는 \(0<\alpha,\beta\), \(\alpha+\beta<90^\circ\)일 때 다른 방법으로도 보일 수 있습니다.

\(\overline{\mathrm{AB}}=1\)이고 직사각형 \(\square\mathrm{AXYZ}\)에 내접하는 \(\angle\mathrm{ACB}=90^\circ\)인 직각 삼각형 \(\triangle\mathrm{ABC}\)입니다.
$$\angle\mathrm{XAC}+\angle\mathrm{XCA}=\angle\mathrm{XCA}+\angle\mathrm{YCB}=90^\circ$$
$$\therefore\angle{BCY}=\alpha$$
$$\angle\mathrm{ABZ}=\angle\mathrm{BAX}=\alpha+\beta$$
$$\overline{\mathrm{AC}}=\cos\beta,\,\overline{\mathrm{BC}}=\sin\beta$$
$$\begin{aligned}\overline{\mathrm{AX}}&=\overline{\mathrm{AC}}\sin\alpha\\&=\cos\beta\cos\alpha\end{aligned}$$
$$\begin{aligned}\overline{\mathrm{BY}}&=\overline{\mathrm{BC}}\sin\alpha\\&=\sin\beta\sin\alpha\end{aligned}$$
$$\begin{aligned}\therefore\cos(\alpha+\beta)&=\overline{\mathrm{BZ}}\\&=\overline{\mathrm{AX}}-\overline{\mathrm{BY}}\\&=\cos\alpha\cos\beta-\sin\alpha\sin\beta\end{aligned}$$
$$\therefore\cos(\alpha+\beta)=\cos\alpha\cos\beta-\sin\alpha\sin\beta$$
$$\begin{aligned}\overline{\mathrm{CX}}&=\overline{\mathrm{AC}}\sin\alpha\\&=\cos\beta\sin\alpha\end{aligned}$$
$$\begin{aligned}\overline{\mathrm{CY}}&=\overline{\mathrm{BC}}\cos\alpha\\&=\sin\beta\cos\alpha\end{aligned}$$
$$\begin{aligned}\therefore\sin(\alpha+\beta)&=\overline{\mathrm{AZ}}\\&=\overline{\mathrm{CX}}+\overline{\mathrm{CY}}\\&=\sin\alpha\cos\beta+\cos\alpha\sin\beta\end{aligned}$$
$$\therefore\sin(\alpha+\beta)=\sin\alpha\cos\beta+\cos\alpha\sin\beta$$
\(\sin(\alpha+\beta)\)와 \(\cos(\alpha+\beta)\)에 대해 같은 식을 얻었습니다.